SPACEGASS Assignment CVEN90049 Structural Theory and Design 2
{`
Department of Infrastructure Engineering
University of Melbourne
CVEN90049 Structural Theory and Design 2
SpaceGass Assignment
`}
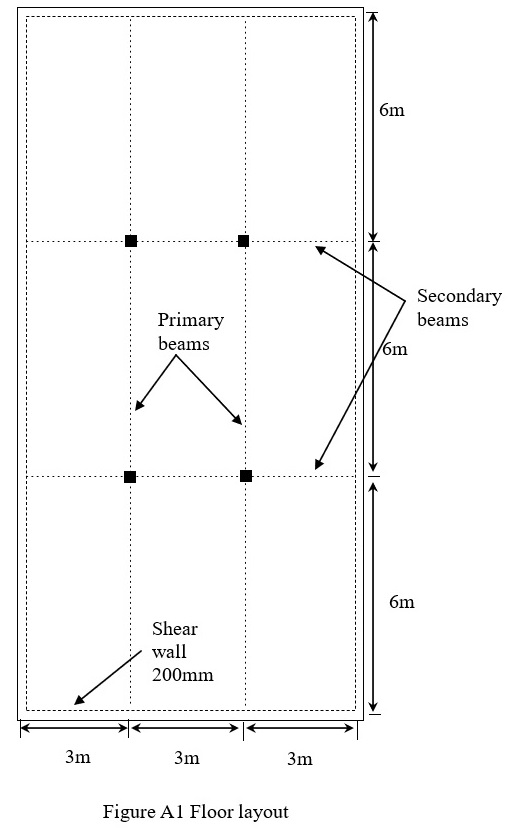
A four-storey office has the general layout shown in Fig. A1in the Appendix A. The floor is to be built of 32 grade reinforced concretes lab. In addition to the self-weight of the structural members, a superimposed dead load of 1kPa should be allowed for. The live load rating of the office floor is 3kPa.The slab thickness is 180mm.
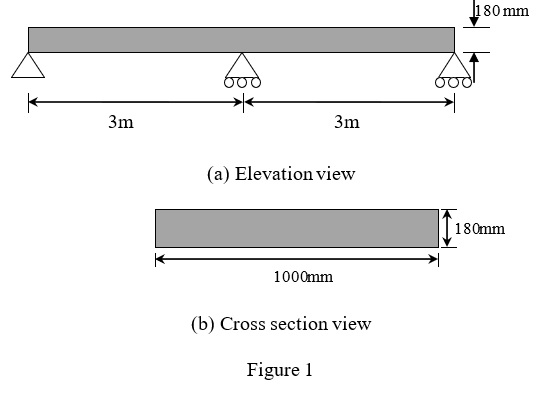
Task 1 To familiarise yourself with the modelling of a continuous beam, build a beam with two continuous spans as shown in Fig. 1 below.
1.Determine the maximum bending moment and reaction force values of the continuous beam under a uniformly distributed load of 10kN/m.
2.Validate the model by comparing the bending moment and reaction force values from the computer analysis with results from manual calculation.
Hint, the beam is indeterminate and hence cannot be solved by simply taking equilibrium conditions. The flexibility (or force) method is probably the easiest way to deal with this problem. The following formula may be useful:
∆ = 5/384 wl4/EI for a simply supported beam subjected to uniformly distributed load w
∆ = 1/48 pl3/EI for a simply supported beam subjected to a point load Pat midspan
Task 2
Use SpaceGass analysis to determine the maximum bending moment and shear force values of the reinforced concrete slab shown in Fig. A1. For the purpose of analysis and design, the slab can be idealised as a continuous beam with 1000mm width shown schematically in Fig. 2.
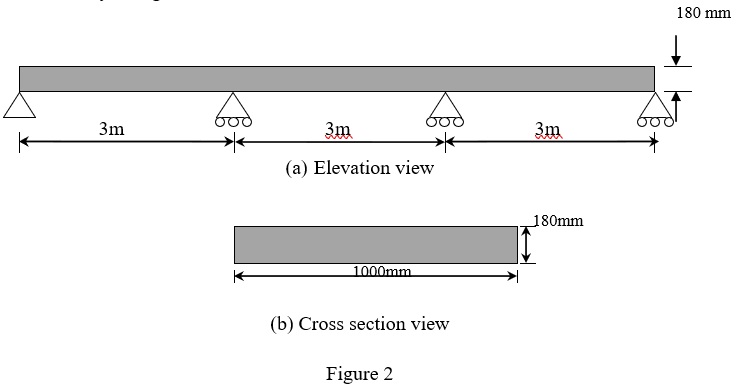
The following load cases apply:
Case 1: 1.2G + 1.5Q
Case 2: 0.8G
Load cases 1 and 2 can be applied at different spans to obtain the maximum values.
Provide a summary of the maximum values for the bending moment and shear force at critical locations and identify the load combinations which produce these values. The critical locations for the bending moment value sareat the supports and at approximately midspans of the continuous beam. The critical locations for the shear force values are at the four supports of the continuous beam.
Compare the maximum values for bending moment and shear force obtained from the Space Gass analysis with the design values according to the simplified method for reinforced continuous beams and one-way slabs in the Australian Standards AS3600(2009) cl. 6.10.
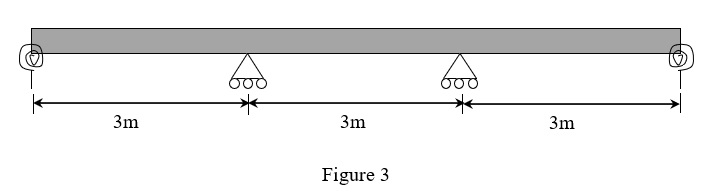
Task 3A S3600(2009)recommends a negative design moment value of FdLn2/24 at the exterior supports of slabs that are supported by beams, when the slabs are built integrally with their supports. This recommendation is based on partial fixity at the exterior supports.
Modify your SpaceGass model for Task 2to account for this partial fixity at the exterior supports. The partial fixity can be modelled by changing the node restraints at the exterior supports into springs with rotational stiffness as shown schematically in Fig. 3.
Calibrate by trial and error the rotational stiffness values of the springs such that the value of the maximum negative moment at the exterior supports matches with that recommended by the standard. Provide a summary of the maximum values for bending moment and shear force at the critical locations.
Comment on the effects of adjusting the restraints on the values of bending moment and shear force at the critical locations.
Report:
Your report should include aim and objectives of the exercise, method, results and discussion, and concluding remarks, addressing the following items:
- Findings from Tasks 1 to 3.
- Your recommendations on the design values for bending moment and shear force at the critical locations(i.e. at the four supports and midspans of the continuous beam).The recommendations should be made based on your Space Gass analysis results.
- Evidence of your analysis models using SpaceGass. The evidence of analysis can be provided by print screen of the models and diagrams of output from SpaceGass. It is not required to include the SpaceGass report in the submission.
The report should not be more than 8pages long.
Appendix A