Statistics Assignment Help With Weibul Distribution

The Weibull distribution is a continuous probability distribution that is commonly used to model the distribution of lifetimes or durations of various events or phenomena. It is often used in reliability engineering and survival analysis. The probability density function (PDF) of the Weibull distribution is given by:

f(x; λ, k) = (k/λ) * (x/λ)^(k-1) * e^(-(x/λ)^k)

Where:

• x: The random variable (usually representing time or duration).
• λ (lambda): Scale parameter, which determines the scale of the distribution.
• k: Shape parameter, which determines the shape of the distribution.

The cumulative distribution function (CDF) is the integral of the PDF and is given by:

F(x; λ, k) = 1 - e^(-(x/λ)^k)

Mean and Variance: The mean (μ) and variance (σ^2) of the Weibull distribution can be expressed in terms of the scale (λ) and shape (k) parameters:

Mean (μ) = λ * Γ(1 + 1/k) Variance (σ^2) = λ^2 * [Γ(1 + 2/k) - (Γ(1 + 1/k))^2]

Where Γ represents the gamma function.

Median: The median of the Weibull distribution can be calculated as:

Median = λ * (ln(2))^(1/k)

Mode: The mode of the Weibull distribution occurs at the value of x where the PDF is maximized. It is given by:

Mode = λ * [(k-1)/k]^(1/k)

Reliability: In reliability analysis, the Weibull distribution is often used to model the failure rate of a system. The reliability function R(x) represents the probability that a system will operate without failure until time x, and it is given by:

R(x) = e^(-(x/λ)^k)

Hazard (Failure) Rate: The hazard rate or failure rate h(x) represents the instantaneous rate of failure at time x, given that the system has survived until time x. It is given by:

h(x) = (k/λ) * (x/λ)^(k-1)

These are some key statistics and properties of the Weibull distribution. Keep in mind that the behavior of the distribution can vary greatly depending on the values of the scale and shape parameters (λ and k). Different parameter values can lead to distributions with different shapes, such as exponential, bathtub curve, or decreasing hazard rate distributions.

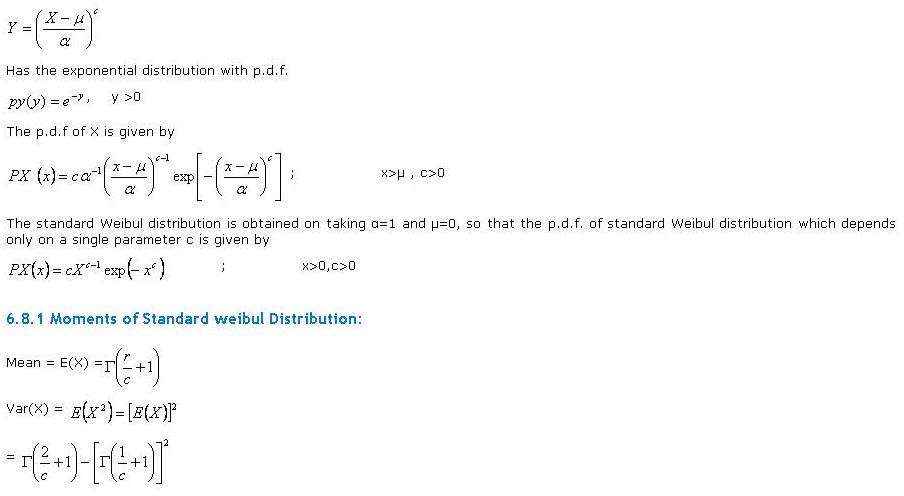
A random variable X has a weibul distribution with three parameters c(>0) and µ if the r.v.

Following are some of the topics in Theoretical Continuous Distributions in which we provide help: